sunnuntai 23. maaliskuuta 2014

Planck aika ja pituus

Kvanttiteorian isä Max Planck (1858-1947) vuonna 1933
kuva wikimedia

Fysiikassa mitataan mikroaikaa yksiköllä nimeltä Planck aika, joka on noin 10−43 sekuntia. Maailmankaikkeuden alussa on ensimmäinen "Planck sekunti".

Näin pieni sekunnin murto-osa on hieman hankalia kirjoittaa nollin tai hahmottaa suhteessa sekuntiin (one onethousand... two onethousand...).

0.0000000000000000000000000000000000000000001 s


Planck aika
wikipedia kertoo
In physics, the Planck time (tP) is the unit of time in the system of natural units known as Planck units. It is the time required for light to travel, in a vacuum, a distance of 1 Planck length. The unit is named after Max Planck, who was the first to propose it.

The Planck time is defined as:
t_P \equiv \sqrt{\frac{\hbar G}{c^5}} ≈ 5.39106(32) × 10−44 s
where 
:\hbar = h / 2 \pi is the reduced Planck constant (sometimes h is used instead of \hbar in the definition [)
G = gravitational constant
c = speed of light in a vacuum
s is the SI unit of time, the second.
The two digits between parentheses denote the standard error of the estimated value.

One Planck time is the time it would take a photon traveling at the speed of light to cross a distance equal to one Planck length.

Theoretically, this is the smallest time measurement that will ever be possible, roughly 10−43 seconds.

Within the framework of the laws of physics as we understand them today, for times less than one Planck time apart, we can neither measure nor detect any change.

As of May 2010, the smallest time interval uncertainty in direct measurements is on the order of 12 attoseconds (1.2 × 10−17 seconds), about 3.7 × 1026 Planck times.
lue koko artikkeli wikipediasta

Planck pituus
wikipedia kertoo
In physics, the Planck length, denoted ℓP, is a unit of length, equal to 1.616199(97)×10−35 metres.

It is a base unit in the system of Planck units, developed by physicist Max Planck.

The Planck length can be defined from three fundamental physical constants:

  • the speed of light in a vacuum, 
  • Planck's constant, and 
  • the gravitational constant.

The Planck length is defined as
\ell_\text{P} =\sqrt\frac{\hbar G}{c^3} \approx 1.616\;199 (97) \times 10^{-35} \mbox{ m}
where c is the speed of light in a vacuum, G is the gravitational constant, and \hbar is the reduced Planck constant. 

The two digits enclosed by parentheses are the estimated standard error associated with the reported numerical value.
lue koko artikkeli wikipediasta


Lähettänyt klo

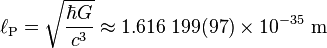
Ei kommentteja:

Lähetä kommentti

Tilaa: Lähetä kommentteja (Atom)